Quadratic Equation with Two Solutions

We can solve a quadratic equation by factoring, completing the square, using the quadratic formula or using the graphical method.

Compared to the other methods, the graphical method only gives an estimate to the solution(s). If the graph of the quadratic function crosses the x-axis at two points then we have two solutions. If the graph touches the x-axis at one point then we have one solution. If the graph does not intersect with the x-axis then the equation has no real solution.

We will now graph a quadratic equation that has two solutions. The solutions are given by the two points where the graph intersects the x-axis.

Example:

Solve the equation x² + x – 3 = 0 by drawing its graph for –3 ≤ x ≤ 2.

Solution:

Rewrite the quadratic equation x² + x – 3 = 0 as the quadratic function y = x² + x – 3

Draw the graph for y = x² + x – 3 for –3 ≤ x ≤ 2.

The solution for the equation x² + x – 3 can be obtained by looking at the points where the graph y = x² + x – 3 cuts the x-axis (i.e. y = 0).

The graph y = x² + x – 3, cuts the x-axis at x = 1.3 and x = –2.3

So, the solution for the equation x + x –3 is x = 1.3 or x = –2.3.

Recall that in the quadratic formula, the discriminant b² – 4ac is positive when there are two distinct real solutions (or roots).

Graphing Quadratic Equations

ax² + bx + c + 0

A Quadratic Equation in Standard Form, (a, b, and c can have any value, except that a can’t be 0.)

is an example:

5x² – 3x + 3 = 0

Graphing

The simplest Quadratic Equation is f(x) = x²

And its graph is simple too:

This is the curve f(x) = x², It is a parabola.

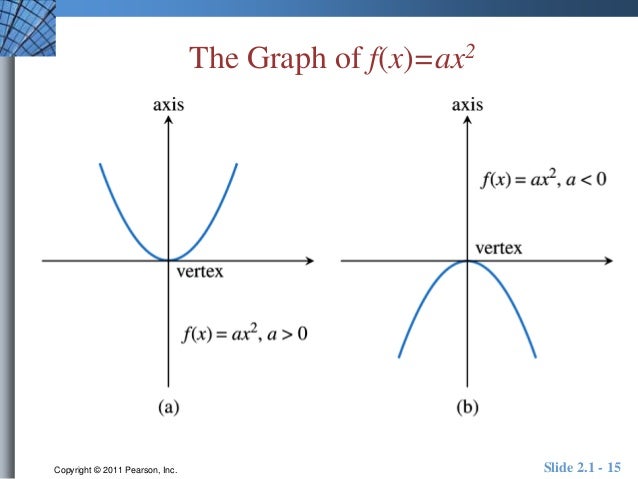
Now let us see what happens when we introduce the “a” value: f(x) = ax²

Let us see an example of how to do this:

Example: Plot f(x) = 2x² – 12x + 16

First, let’s note down:

a = 2, b = -12, and c = 16

Now, what do we know? a is positive, so it is an “upwards” graph (“U” shaped), a is 2, so it is a little “squashed” compared to the x² graph

Next, let’s calculate h:

h = -b/2a = -(-12)/(2×2) = 3

And next we can calculate k (using h=3):

k = f(3) = 2(3)² – 12·3 + 16 = 18-36+16 = -2

So now we can plot the graph (with real understanding!):

Assessment:

1. From the above graph to factor out the roots.
   x²– 9x + 20 B. 2x²– 9x + 20 C. x² – x + 20 D. x² – 9x + 9
2. What makes this equation 2x² – 12x + 16 quadratic?
3. The coefficient of x²B. The coefficient of x which is 12  C. The squared power of x whose coefficient is 2   D. None of the options is correct.
4. What is a parabola?
   A. The x – axis  B. y – axis C. The curve on the graph D. None of the options.
5. a < 0 and in the quadratic equation, means that the curved end parabola is Up on the graph B. Down on the graph C. Left side on the graph  D. Right side on the graph.