Speed

The speed of an object is a measure of how fast something is traveling.

speed (m/s) = distance (m) ÷ time (s)

Alternatively the units are kilometers per hour (km/h).

You will also see miles and miles per hour:

• 1 mile = 1.6 km = 1600 m
• 1 mph = 0.44 m/s

Despite this country’s love of imperial distance measurements, all Highways and many main roads have distance markers in kilometers.

Most modern engineering is done entirely metrically, and has been for many years.

The equation can be rearranged to:

distance = speed × time

or

time taken = distance ÷ time

OR:

1. Distance (s)

This is the separation or space between two points. It is measured in meters and it is a scalar quantity.

2. Displacement (s)

It is distance in a specified direction. It is a vector quantity and it is measured in meters.

3. Speed (v)

It is the rate of change of distance moved with time. The unit is m/ and it is a scalar quantity.

Speed=distancetimev=st

(a) Uniform speed

It is obtained if the rate of change of distance with time is constant or when a body travels equal distances in equal time intervals.

(b) Average speed

Average speed is the total distance travelled divided by the total time taken. The average speed is a better representation of the motion of a body not moving at a constant or uniform speed.

Average speed=Total distance coveredTotal time taken

(c) Instantaneous speed

It is the actual speed of a body at any instant during the course of motion.

4. Velocity

It is the rate of change of displacement with time. The unit is m/s. It is avector quantity.

Velocity=displacementtime

Uniform Velocity

It occurs when the rate of change of displacement with

time is constant or when a body travels equal displacement in equal time interval.

EVALUATION

1. Define speed, velocity and uniform velocity.
2. Differentiate between velocity and speed.

Calculations on Speed and Velocity

1. A car covers a distance of 60km in half an hour. What is the average speed of the car in (a) km/hr  (b) m/s

Solution:

(a) Time = ½ hour = 0.5 hour =12hour=0.5hour

Average speed=Total distance coveredTotal time taken=600.5=120km/h

(b) Convert  km/hr to m/s

1km/h=100060×60m/s1km=1000m1hr=3600s120km/h=120×100060×60=33.33m/s

2. A car travelled to Lagos a distance of 150m in 100 seconds. Calculate his average speed.

Solution:

Average speed=Total distance coveredTotal time taken=150100=1.5m/s

3. A car covers 1500m in 10 secs. What is the speed in km/hr?

Solution:

Speed=distancetime=150010=150m/s

Convert to km/hr

1km/h=100060×60m/s1m/s=60×601000km/h150m/s=150×60×601000=540km/h

EVALUATION:

1. Convert 144km/h to m/s.
2. A car covers a distance of 40m in 2 sec. What is his speed in km/h? 

Distance-Time Graph, Displacement-time Graph

It is the graphical representation of the motion of a body. There are Distance-time graph, Displacement-time graph and Velocity-time graph.

(a) Distance-Time Graph for Uniform Motion

distance time graph

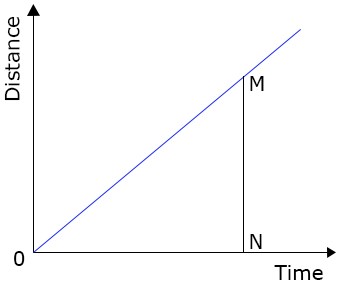
Slope of distance–time graph = speed

Distance-Time Graphs and Velocity-Time Graphs:

https://www.slideshare.net/slideshow/embed_code/key/qjs9T4EU9KN6baDistance time graphs for Stoplearn.com

The Concept of Acceleration

When an object increases or changes its velocity within a set time, the object is said to undergo acceleration (or to accelerate). We therefore define acceleration as the rate of change of velocity with time.

Acceleration=Change in VelocityTime takena=v–ut

Where a: acceleration

v: final velocity

u: initial velocity

Acceleration is a vector quantity and its SI unit is m/s2

However, be reminded that:

(i) When a body starts from rest, its initial velocity, ‘u’ is zero.

(ii) When a body comes to rest, its final velocity, ‘v’ is zero.

Uniform and Non-uniform Acceleration

Acceleration is said to be uniform if the velocity increases by equal amounts in equal intervals of time. That is, the time rate of change of velocity is constant. If the rate of change of velocity with time is not constant, then, the acceleration is non-uniform.

Deceleration

Deceleration is defined as a negative change in velocity with time. When such happens, the body’s velocity is said to be reducing or coming to rest.

Deceleration is said to be uniform if the velocity decreases by equal amounts in equal intervals of time. That is, the negative change in velocity with time is constant.

Also, Deceleration=Change in VelocityTime taken=v–ut

Deceleration is also called retardation and its SI unit is m/s². It is also a vector quantity.

EVALUATION

1. Define acceleration.
2. Differentiate between acceleration and deceleration.
3. Quote the formula for acceleration and its SI unit.

Worked Examples on Acceleration and Deceleration

Example 1: A body experienced a change in velocity of 10m/s in 15s. What is the acceleration of the body?

Solution:

Data: ∆v = 10m/s, t = 15s, a = ?

Now, a=ΔvΔt=1015=0.67m/s2

Example 2: A car accelerated uniformly at 6m/s² in 20s. What was the change in velocity?

Solution:

Data: a = 6m/s², t = 20s, ∆v = ?

Now, a=ΔvΔtΔv=a×ΔtΔv=6×20Δv=120m/s

Example 3: The velocity of a lorry decreased from 60km/h to 35km/h within 0.5mins. Find the deceleration.

Solution:

Data: u=60kmh=60×100060×60=60003600=16.67m/s,v=35km/h=9.72m/s,t=0.5mins=30s,d=?

Now, d=ΔvΔt=v–ut=9.72–16.6730=−6.9530d=−0.23m/s2

The negative sign shows that it is decelerating thus coming to rest.

( NOTE: you can convert velocity in km/h to m/s by simply dividing by 3.6 )

EVALUATION

1. Find the deceleration of a car whose change in velocity within a time interval of 10s is -30m/s.
2. A car started from rest and accelerates uniformly until it reaches a maximum velocity of 80km/h in 20s. It is then brought to rest in further 12s. Find the deceleration of the car.

GENERAL EVALUATION

1. What is the similarity between acceleration and deceleration?
2. Differentiate between acceleration and deceleration.
3. Write down the formula for acceleration.

EVALUATION

1. A car starts from rest and accelerates uniformly at  until it attains a maximum velocity of  in 10s. It continues with this speed for further 100s until it is brought to rest for another 25s. Using a v-t graph, find the total distance travelled.
2. A body moves with a constant speed of 10m/s for 10 s then decelerate uniform to rest in another 5 s. (i) Draw a velocity–time graph to illustrate this motion. (ii) calculate the deceleration of the body (iii) calculate the total distance travelled by the body.

N.B: for bodies moving with constant or uniform acceleration, the following formulas could be used.

v=u+at… … … (i)

s=ut+12at2… … … (ii)

s=(v+u2)t… … … (iii)

v2=u2+2as … … … (iv)

Worked Examples

1. A body starts from rest and accelerates at until it gets to a final velocity in 20s. It is then brought to rest in another 10s. Using equations, find the final velocity attained and the total distance travelled.

Solution:

1st stage data: u = 0 (because it started fron rest); a = 2m/s2, t = 20s; v = ?

2nd stage data: u = value of ‘v’ above; v = 0 (because it came to rest); t = 10s

1. i) Using v = u + at v=u+at

∴ v=0+2×20=0+40

∴ v=40m/s

1. ii) To get the total distance covered, let’s get the distance covered in each stage.

In the first stage, using v2=u2+2as

∴ s =v2–u22a=402–02×2=16004=400m

In the second stage, using v2=u2+2as

Here, “a” is deceleration and not acceleration. We need to find it.

Deceleration =v–ut=0–4010=−4m/s2

∴ s=v2–u22a=0–4022×−4=−1600−8=200m

∴ Total distance travelled =400m+200m=600m

(NB: You can also use s=ut+12at2 for each stage)

2. A car starts from rest and accelerates uniformly at until it reaches a final velocity of in 23s. It is then brought to rest for further 15s. Using equations,

Find the value of x
Find the distance travelled during the acceleration and the deceleration.
Find the total distance travelled.

Solution:

1st stage data: u = 0 (Because it started from rest); a = xm/s²; v = 60m/s, t = 23s.

2nd stage data: u = 60m/s; v = 0 (because it came to rest); t = 15s

232. i) Using a=v–ut=60–02361m/s2

∴ x=2.61m/s2

1. ii) Distance covered during the acceleration, using v2=u2+2as

s=v2–u22a=602–02×2.61=36005.22=689.6m=690m

Now, the deceleration =v–ut=0–6015=−4m/s2

Now, s=v2–u22a=0–6022×−4=−3600−8=450m

Total distance travelled =690m+450m=1,140m.

E=MC2

Speed, Distance and Time

1. Work out the speed of a car traveling:
2. 100m in 10s
3. 300m in 20s
4. 700m in 35s
5. 1000m in 200s

2. How far does a lorry move if it’s traveling at:
3. 10m/s for 30s
4. 20m/s for 20s
5. 2m/s for 100s
6. 15m/s for 28s

3. How long does it take a car to travel:
4. 10m at 20m/s
5. 50m at 10m/s
6. 200m at 8m/s
7. 50km at 100km/h

4. Car A travels 100m in 5 seconds. If car B travels the same distance in 2 seconds, which one is traveling at the faster speed? Explain your answer.

5. Look at the table below, showing the time taken to travel a distance of 100m by 5 different pupils in a year 11 class:

Name	Time taken (s)
Jenny	15
Ahmed	12
Kevin	13
Julia	12
Louise	16

1. Who traveled at the fastest speed?
2. Who traveled at the slowest speed?